Universal Cost Bound of Quantum Error Mitigation Based on Quantum Estimation Theory

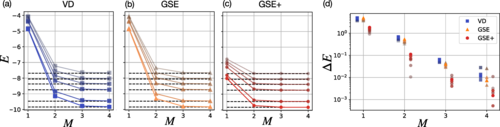
We present a unified approach to analyzing the cost of various quantum error mitigation methods on the basis of quantum estimation theory. By analyzing the quantum Fisher information matrix of a virtual quantum circuit that effectively represents the operations of quantum error mitigation methods, we derive for a generic layered quantum circuit under a wide class of Markovian noise that, unbiased estimation of an observable encounters an exponential growth with the circuit depth in the lower bound on the measurement cost. Under the global depolarizing noise, we in particular find that the bound can be asymptotically saturated by merely rescaling the measurement results. Moreover, we prove for random circuits with local noise that the cost grows exponentially also with the qubit count. Our numerical simulations support the observation that, even if the circuit has only linear connectivity, such as the brick-wall structure, each noise channel converges to the global depolarizing channel with its strength growing exponentially with the qubit count. This not only implies the exponential growth of cost both with the depth and qubit count, but also validates the rescaling technique for sufficiently deep quantum circuits. Our results contribute to the understanding of the physical limitations of quantum error mitigation and offer a new criterion for evaluating the performance of quantum error mitigation techniques.